6.2 ANSWERS normal path
Answers exercises
-
Exercise 1:
Given the formula y = -x2
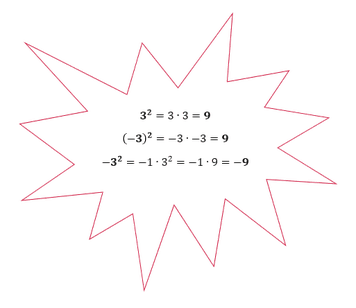
a) Calculate y for x = 3
y = -32
y = -3 . 3
y = -9
b) Calculate y for x = -4
y = - (-4)2
y = - ( -4 . -4 )
y = -16
-
Exercise 2:
Given the formula Q = -( 2l + 4 )2
a) Calculate Q for l = 1
Q = -( 2 . 1 + 4 )2
Q = -( 2 + 4)2
Q = -62
Q = -36
b) Calculate Q for l = -3
Q = -( 2 . -3 + 4 )2
Q = -( -6 + 4)2
Q = -(-2)2
Q = -4
c) Calculate l for Q = -144
-144 = -( 2l + 4 )2
Two methods:
- -144 = -( 2l + 4 )2
Just try some numbers to find the correct answer. You will see that l = 4 perfectly fits the given formula.
Or
- -144 = -( 2l + 4 )2
first divide everything by minus one because of the minus symbol.
144 = ( 2l + 4 )2
Take the square root to get rid of the squared.
√144 = 2l + 4
12 = 2l + 4
All numbers to one side and all letters to the other side. So, you need to do -4 on both sides.
8 = 2l
Divide everything by the number before the letter. So, you need to divide everything by 2.
4 = l
l = 4
-
Exercise 3:
Robin is playing tennis. For one of his serves the height h of the ball is given by the formula:
h = -0.1d2 + 1.2
where h is the height in meters and d the distance in meters.
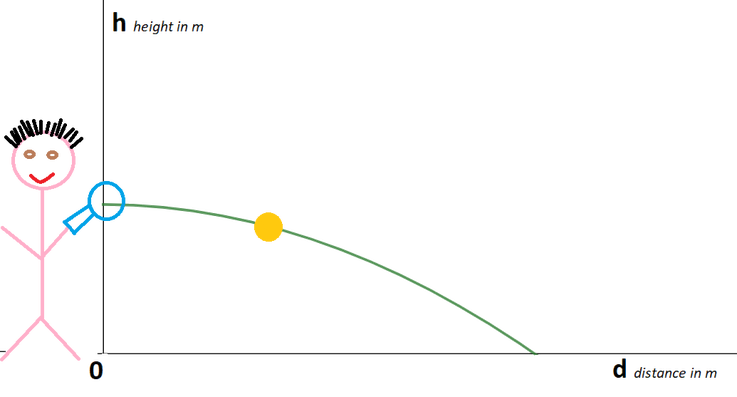
a) Calculate the height of the ball for d=2
h = -0.1d2 + 1.2
d= 2 so, fill it in.
h = -0.1 . 22 + 1.2
h = -0.1 . 4 + 1.2
h = -0.4 + 1.2 = 0.8 m
So, the height of the ball at a distance of 2 meters is 0.8 meters.
b) What is the height of the ball at the start?
The distance is 0 meters at the start.
h = -0.1 . 02+ 1.2
h = 0 + 1.2
h = 1.2
So, the height of the ball at the start is 1.2 meters.
c) At what distance does the ball hit the floor?
The ball hits the floor at a height of 0 meters.
0 = -0.1d2 + 1.2
all numbers to one side and all letters to the other side.
0.1d2 = 1.2
Divide everything by the number before the letter. So, divide everything by 0.1.
d2 = 12
d= √12 ≈ 3.5 meters
So, the ball hits the floor at a distance of 3.5 meters



